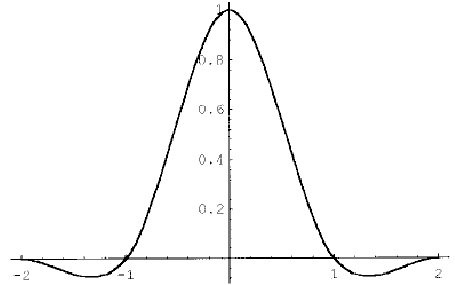
 [A1]
[A1] 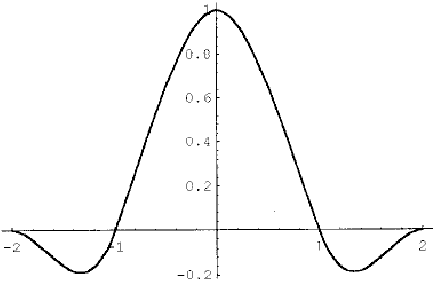 [B1]
[B1]MTF Performance for Image Resampling from a 1/3-; 2/3-; 4/3-km Input Grid to a Standard 1/2-; 1-; 2-km Output Grid
A1. Interpolation Function 1---------------------------- B1. Interpolation Function 2
The horizontal scale is in units of the spacing of the source pixel. The 1-dimensional interpolation is over 5 pixels. The resampling for a 2-dimensional image is done in two 1-dimensional steps. The first interpolation might be for the spacing in the scan direction along rows of pixels. The second interpolation is of the resampled rows in the direction perpendicular to the scan direction to get the interpolation between rows.
Interpolation Function 1 is a "cubic convolution" algorithm developed for LANDSAT. Interpolation Function 2 was arbitrarily selected to provide an MTF of 1 at the resampled Nyquist frequency and 1 or higher between 0 and the Nyquist frequency. In example B, the resampling improves MTF performance at the cost of increasing noise in the resampled data.
The "A" value in the charts below is the alignment offset of the Interpolation Function in fractional pixel spacing between source pixels: 0 being with the peak exactly aligned with the source pixels; 0.125 being shifted by 1/8th pixel; to 0.5 being spaced half way between source pixels. The 0.5 spacing is the worst case. The k (cy/input pixel) value of 0.5 corresponds to 1 cycle for two pixels, the definition of the Nyquist frequency. Comparing 1/3- with 1/2-km/pixel the Nyquist frequency in output space is 0.67 times the input space frequency or 0.5 x 0.67 = 0.33. Therefore, 0.5 corresponds to 1/3-km pixels, 0.33 to 1/2-km pixels. The same ratios apply to the conversion from 2/3-km to 1-km pixel spaces and from 4/3-km to 2-km pixel spacing.
A2. MTF in Input Space for Interpolation Function 1--------- B2. MTF in Input Space for Interpolation Function 2
A3. MTF in Output Space for Interpolation Function 1 B3. MTF in Output Space for Interpolation Function 2
In the "A" example, the average MTF decrease is to about 0.85 at the 1/2-km output Nyquist frequency, 0.68 at the lowest. For the "B" resampling, there is a slight improvement in MTF performance because of resampling. The "B" procedure is known to increase noise. The "A" case is a result of working with LANDSAT images so it is likely we might chose similar interpolation.
(Interpolation functions & graphs provided by Jim Carr of Carr Astronautics Corporation.)